I am reading a paper where the authors made the following statement on page 16:
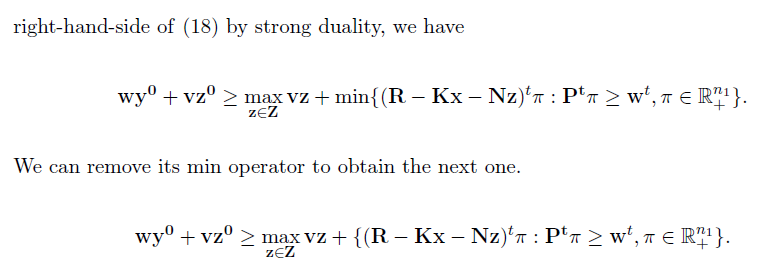
I wonder why dropping the 'min' operator inside a 'max' objective function is a valid operation here. Any explanation with simple example will be highly appreciated.
I am reading a paper where the authors made the following statement on page 16:
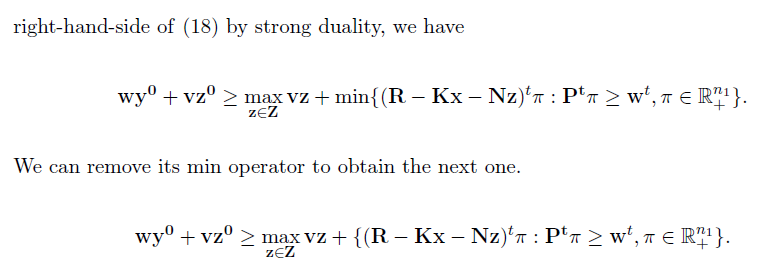
I wonder why dropping the 'min' operator inside a 'max' objective function is a valid operation here. Any explanation with simple example will be highly appreciated.
A simple intuition would be that the second inequality is stronger ($\geq$) than the first one. Therefore, dropping the minimum would allow more flexibility, but I do not think they are equivalent.