Units in the EOQ problem

Question

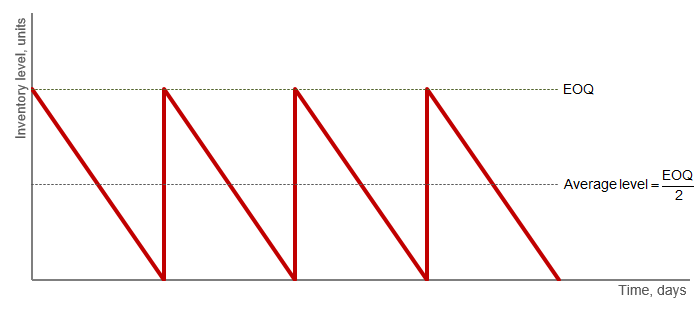
This is a very basic question about a very basic model, but I can't come up with a satisfactory answer.

In the economic order quantity (EOQ) model, let $\lambda$ be the demand rate (items/year), $h$ be the holding cost (\$/item/year), and let $K$ be the fixed cost per order. The optimal solution is $$Q^* = \sqrt{\frac{2K\lambda}{h}}.$$

What should be the units for $K$? One option is just \$, which would give $Q^*$ units of $$\sqrt{\frac{\$ \cdot \frac{\text{item}}{\text{year}}}{\frac{\$}{\text{item}\cdot\text{year}}}} = \text{item},$$ which is right.

But it seems equally plausible to me to use \$/order as the units for $K$, but then we have units $$\sqrt{\frac{\frac{\$}{\text{order}} \cdot \frac{\text{item}}{\text{year}}}{\frac{\$}{\text{item}\cdot\text{year}}}} = \text{item}\sqrt{\frac{1}{\text{order}}},$$ which obviously doesn't make sense. It would also work out if there were another $\sqrt{1/\text{order}}$ somewhere, so that $Q^*$ has units $\text{item}/\text{order}$, but none of the other terms should have $\text{order}$ in their units.

What am I missing?

Answer 1

In the EOQ setting, the total cost incurred during one order cycle is: $$TC = K + \frac{hQ^2}{2 \lambda} \;\;,$$ where the units of $K$ must be only \$ and $Q$ measures the inventory count in items after placing the order at the beginning of the cycle. If $K$ were to have units of \$ per order (or per cycle), then the second term must also have those units otherwise you are adding apples to oranges. Given $TC$ measured only in \$, we complete the model and compute $\frac{TC}{t}$ by dividing by the cycle length of $\frac{Q}{\lambda}$ and then find $Q^*$ to minimize cost per time in the right units of items.

One possibly important idea is that there are only three fundamental dimensions in the EOQ model, where a dimension is a quantity that must be measured to specify the model. The three fundamental dimensions are cost (typically measured in \$), time (measured in years), and inventory (measured in items). In my setup above, $TC$ is a cost variable and is comprised of two cost components, both measured in \$. So $K$ has units of \$ and provides the fixed cost incurred in an order cycle. The second term has $h$ measured in \$ per item-year multiplied by $\frac{Q^2}{2 \lambda}$ measuring average item-years of inventory in an order cycle since the average inventory $\frac{Q}{2}$ is measured in items and the cycle length $\frac{Q}{\lambda}$ is measured in years assuming an initial inventory of $Q$ items. In the EOQ setup, constant order quantities makes it unnecessary to measure orders separately to specify the problem. If the unit of orders is called for simplicity unit order, then the unit orders per time is just an endogenous performance metric given by demand (items per year) divided by order size (items per unit order), or $\frac{\lambda}{Q}$. Note that it is perfectly fine for $Q$ to be a quantity of items or a quantity of items per unit order, depending on the setting.

If you are still wanting more, it is also possible to guess wrong and assume that it is necessary to measure orders explicitly when specifying a correct EOQ model. Let's think about this. Let $K$ in this case be a cost per order, measured in \$ per unit order. We can furthermore define the variable $Q$ as measuring inventory per order, the increase in inventory obtained for every order measured in items per unit order. Let me also suggest that variable quantity $o_t$ captures the orders placed at time $t$ again measured in unit orders. Consider a time duration that begins with a time $t$ when an order is placed. It isn't difficult to see that there should be no items in inventory at $t$ for an optimal ordering strategy. If we place $o_t$ unit orders, the inventory level will be raised to $Q o_t$ at a cost of $K o_t$. During the time interval while the inventory again drops to zero, the total cost will be: $$TC' = K o_t + h \frac{Q o_t}{2} \frac{Q o_t}{\lambda} \; ,$$ where again both terms measure cost in \$ (the second term is again \$ per item-year multiplied first by items and then by years). Dividing again by the length of the time interval yields: $$\frac{TC'}{t} = \frac{K \lambda}{Q} + h \frac{Q o_t}{2} \; .$$ Both terms measure cost correctly in \$ per year, but it should be clear that cost only increases in the decision variable $o_t$ so it should be set equal to one unit order before optimizing for $Q$. In this formulation, it is true that $Q$ (and $Q^*$) is in units of items per unit order whereas in the original formulation $Q$ simply measures the increase in inventory that results by placing the single order. Since only one unit order is placed in a reorder cycle, we strip away the need to have $K$ and $Q$ measure per unit order quantities.

I'm sorry for the long answer, but I hope it helps.

Answer 2

The coefficient $2$ in the first equation has unit $1/\text{order}$, so the second approach is the right one, and $Q^*$ has units $\text{item}/\text{order}$.

The unit comes from the holding cost $hQ/2$ in the formula for the total cost, which assumes that for order quantity $Q$ (items/order) you have in average $1/2$ order in stock, so $1/2$ has unit "order", and $hQ/2$ is in $\$/\text{y}$.

Answer 3

It is an interesting question.

EOQ model starts from that the minimum point of the total cost (Inventory holding + Ordering cost). At the minimum point, the Inventory holding cost equals to the Ordering cost.
(of course you can use calculus to find the minimum point but the answer will be the same)

$\frac{Q}{2}h = \frac{\lambda}{Q}K$

The problem starts from here. $\frac{Q}{2}$ is the average inventory level over the year. Therefore, The unit should be Item. However, $\frac{\lambda}{Q}$ is the number of orders per year . Therefore if the $\lambda$ is Item per Year, Q has to be Item/order.

If we accept $Q$ has the unit Item/order, The average Inventory level $\frac{Q}{2}$ should have the unit, Item/order, but it should be Item for average Inventory level.

the $Q$ in $\frac{Q}{2}$ and $Q$ in $\frac{\lambda}{Q}$ are different in the unit.

1) The average inventory can be calculated by dividing the highest point in the sawtooth shape cycle by 2. The highest point is the highest inventory level, so the unit should be Item.

How do we find the highest point? We know the highest point in the inventory level is the $Q$, order quantity, (Item per order)

They are indeed the same number (but in different units) so that we can solve the equation.

2) The Average Inventory level per order, $\frac{Q}{2}$ (unit: Item/order) is the Annual average Inventory level $\frac{Q}{2}$ (unit: Item)
But when we calculate Annual inventory holding cost, we use 'annual average inventory level', not 'average inventory level per order'.

Not sure it is what you are looking for. Expecting discussions

Answer 4

I remember encountering the same question when teaching Operations Management for the first time.

The thing is, annual total inventory cost is simply total annual ordering cost plus total annual holding cost, all of them measured in $\frac{\$}{\rm year}$. The total ordering costs is simple: number of orders per year times fixed cost per order: $\frac{D \, \rm items/year}{Q \, \rm items/order} \times K \frac{\$}{\rm order} = \frac{\$}{\rm year}$.

The total holding cost can be calculated as annual holding cost per item times average inventory level, which is measured in items and happens to be equal to $\frac{Q}{2}$. Usually, in textbooks they use continuous inventory depletion model, like in the picture below, and use the triangle area formula to explain the intuition.

However, in for more general settings the EOQ formula derivation is even less straightforward, and involves derivative and integration. To explain it in apples-to-apples manner you would have to go deep into mathematical realms, which defeats the whole purpose. I noticed that in textbooks, even the Master level ones, they drop the measurement units issue in discussion of EOQ, newsvendor model and other nonlinear topics.

PS: If I remember correctly, in the undergraduate textbook I was using (Stevenson), $K$ was measured in just dollars.